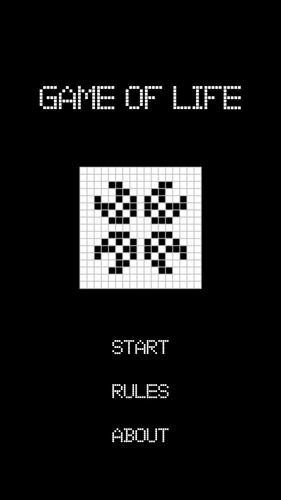
John Conway's Game of Life, a classic cellular automaton, was conceived in 1970. This game unfolds on an infinite, two-dimensional grid where each cell exists in one of two states: alive or dead. The game's evolution, or generation, occurs in discrete steps, with each cell's fate determined by its eight neighboring cells (horizontally, vertically, and diagonally adjacent).
The initial arrangement of live and dead cells forms the first generation. Subsequent generations are produced by simultaneously applying the following rules to every cell:
- Survival: A live cell remains alive if it has exactly two or three live neighbors.
- Birth: A dead cell becomes alive if it has exactly three live neighbors.
Conway experimented with numerous rule variations before settling on this specific set. Other variations often lead to population extinction or uncontrolled expansion. The chosen rules represent a critical point between these extremes, resulting in the complex and fascinating patterns for which the Game of Life is renowned. This delicate balance between expansion and decay creates a rich tapestry of dynamic behavior.
Tags : Simulation